Corner-Mover: UR *7.5
(moves fld to bdl)

Edge-Mover: U2R2 *3
(moves fu to bu, also swaps fr and br)
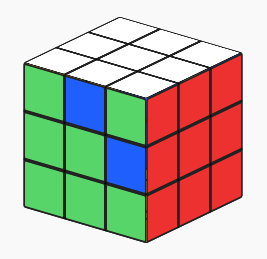
|
Corner-Mover: UR *7.5 (moves fld to bdl) 
|
Edge-Mover: U2R2 *3 (moves fu to bu, also swaps fr and br) 
|
|
First we solve all corners, using one simple move and process. Then we solve all edges, using a very similar move and process. Corner and edge moves are similar in form, function, and application. The 2 moves are simple, symmetric, and repetitive, using only the easy Up and Right turns. The 2 moves have simple, direct, linear, binary effects, and are easy to apply: aim, fire, reset. The entire method can be described to experts in 20 words; 7 words for the 2x2x2 cube. The method is minimal like the game of Go, unary/binary numbers, or Turing Machines. Simple tools and powerful concepts to learn, understand, and then apply creatively. Method, tools, concepts, description, and the creative role of the solver, are all beautiful. |
|
Corners: 1. When we do the corners, we don't yet care about the edges. 2. Insight: If we have a Transform that can move one Corner Cubie into an adjacent Corner Cubicle, then we can use this Transform to move any Corner Cubie into any Corner Cubicle, and thus we can send all the Corners home. 3. We orient Corners as we move them home, by lining them up correctly. Each Corner Cubicle is on 3 edges, and therefore can be approached from 3 corner neighbours. Each approach can be lined up in 3 orientations. 3 of the 9 line-ups will be correct, resulting in the desired orientation, and at least one of them will require at most 2 line-up turns. Thus, we never need more than 2 line-up turns for the corners, which makes it really easy to invert the corner line-up turns after applying the corner-move transform. 4. The Corner-Move Transform cannot twist in place! If we end up with cubies twisted at home, we have to move them away, so we can orient them properly on the way back home. 5. At most 3 applications of the Corner Move Transform are needed for the last 4 corners. 6. The Corner-Move actually moves the other 6 corners! To keep track of the face colors, you can just use the "solved" corners for reference, and disregard the centres. Optionally, for clarity, you can adjust the centres between moves. 7. A 2x2x2 Cube is valuable for getting started, and for learning the Corners. To keep track of the face colours, with no centres, just pick a cubie that is "solved" as a reference. (But this is not really necessary, since at most 2 line-up turns are needed, so it's obvious what to invert.) Edges: 8. When we do the edges, we won't disturb the corners; and we keep the centres aligned. 9. Insight: If we have a Transform to move one Edge Cubie into an opposite Edge Cubicle, we can use this to send every Edge Cubie home, properly oriented. It is simply a matter of correctly lining up a cubie across from its home cubicle, along with a helper pair, applying the transform, and then undoing the line-up turns. 10. There are 4 ways of lining up a displaced edge cubie opposite its home cubicle, 2 sane, 2 flipped. 11. The Edge-Transform employs a Helper Edge-Pair , for which we pick two edges that we don't care about yet, or an already matched pair. (A pair of swapped opposite edges are extremely easy to swap back. They are practically solved.) If it is difficult to find a convenient helper pair, it may be better to send another cubie home first, and do this one later. ( The concept of "Helper Edge-Pair" is similar to a catalyst. It is a manageable side-effect of the Edge-Mover, which actually swaps two edge-pairs.) 12. It takes at most 3 line-up turns to line up an edge cubie. It may take another 1 or 2 turns to line-up a helper pair. So, we only need to start remembering "undo-turns" after 2 turns, since the last 2 undo-turns should be obvious. General: 13. When applying any Transform, always hold the left-down portion of the cube, with left thumb in front and left index and middle fingers in back, so that only Up and Right faces are moveable. 14. Think of a transform as a tool that moves one Cubie into its Home Cubicle, rather than "swaps 2 cubies". We send one cubie home at a time. 15. Always: "Line Up across from Home, Apply Transform to send Home, Undo the Line Up turns." 16. Name and Remember the line-up turns by Colour, not by Position. E.g.: UnRed; BigBlue UnRed; Red BigBlue UnRed. 17. Doing the first 4 corners and the first 4 edges intuitively is merely an optional shortcut. Perhaps not worth mentioning. 18. This method is not fast. It is not efficient. We send one cubie home at a time. There are 20 cubies. At an average of 10 turns per cubie, expect 200 turns. At one turn per second, expect 3-4 minutes of leisurely solving the Cube. 19. Be sure to understand the effects of the transform-tools, how to achieve proper line-up through correct orientation, and how to invert line-up turns, before you try to solve a cube. With the Elegant Method you are in control, so please don't interpret description as procedural instruction that must be followed passively and unthinkingly without understanding. This method is not like those other methods. Perhaps we can say that other methods use many complicated concepts and procedures that make the solution process mechanical, efficient, and thought-free. The Elegant Methed uses only 2 tools and 4 concepts, but requires a little imagination, intuition, and thought for each move. 20. I know of no other Methods that claim to be beautiful, minimal, or elegant. It seems that Philip Marshall's "Ultimate Solution" and the "Old Pochman Method" may be minimal in some ways, but both seem complicated. If anyone knows of another Method that is elegant, minimal, simple, or beautiful, please let me know. Thanks! -- peter [AT] dancing [DOT] org |
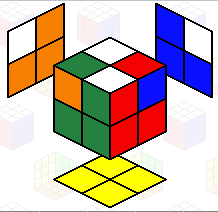
|
First we simply swap the two twisted corners, so that we can orient them later, on the way home; line-up: UnRed; this makes the 2 cubies adjacent, rather than diagonal; put left index finger on Wgo, left thumb on Bwr; (Wgo means the White facelet of the WGO cubie); (The left thumb's facelet will be at Front-left-down for the Corner-Move); hold the cube with those two at left-down; apply corner-move transform UR *7.5; invert: Red. |
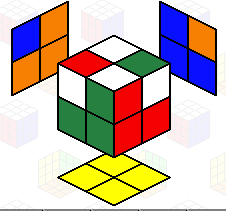
|
Now the two cubies are in each other's place; let's send RWB home; let's line it up with its Red facelet opposite its home, so that the Red facelet will end up on the Red face, after the Corner-Move. line up: UnGreen Big-White; put left index finger on Wgo, left thumb on Rwb, hold the cube with those two at left-down; apply corner-move transform UR *7.5; invert: Big-White Green. -- DONE! |
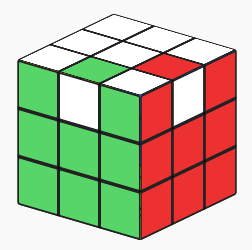
|
Let's just swap the 2 flipped edges; line up: BigRed UnYellow; helper line up: UnRed; hold cube so that RedWhite is at fu, BlueRed is at fr; apply edge move transform U2R2 *3; invert: Red Yellow BigRed; |
|
Now the 2 cubies are swapped; RedBlue and RedGreen helpers are also swapped; let's send GreenWhite home; line up: Red Blue; this aims GreenWhite home; helper line up: UnRed; (conveniently same as before) hold cube so that WhiteRed is at fu, BlueRed is at fr; apply edge move transform U2R2 *3; invert: Red UnBlue UnRed. -- DONE! |
[ Image credits: Jaapsch.Net, Rubiks-Cube-Solver.Com ]
[ peter [at] dancing [dot] org ]